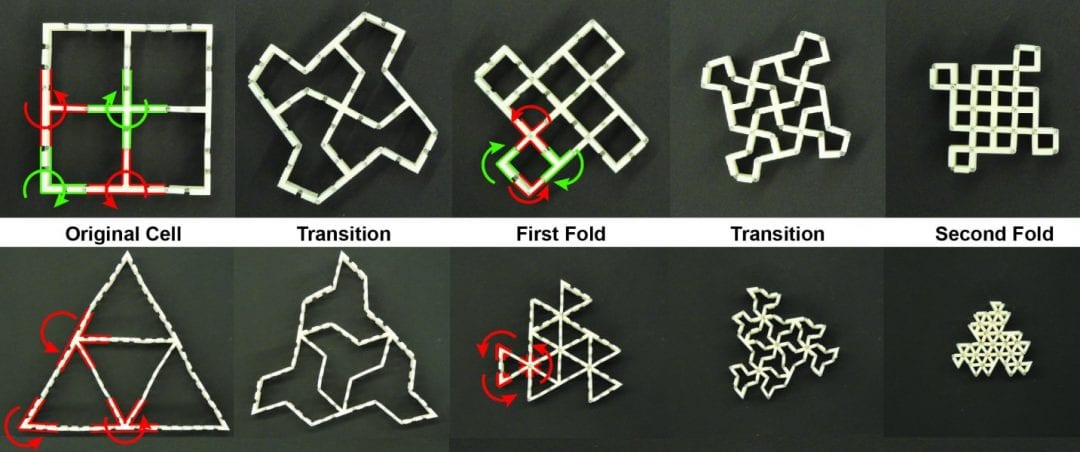
The inherently low volume fraction and ordered tessellated organization of lattice materials can be exploited to obtain significant topological (and thus behavior) changes by folding and unfolding of the individual segments of the lattice wall. The authors introduce a novel self-replicating and reversible folding scheme for lattice materials which relies on the rotation of the wall segments that can be achieved either unassisted or assisted with active actuating units and can be applied on lattices with square and triangular grid topologies. The described folding transitions results in 50 and 67% reduction in the effective area of the square and triangular lattices, respectively, while the design enables multiple levels of folding to achieve a desired final size.

Although area reduction in the folding process does not depend on the direction of rotation of the nodes (i.e., clockwise or counter clockwise), it can affect the shape of the final folded structure. For structures with relatively small numbers of mesh, the sequence of rotation directions will determine the shape of the folded lattice, however, for large lattices the overall shape will be nearly preserved during folding for all combination of rotations (see the Supporting Information). In other words, for an infinite lattice, the final shape does not depend on the sequence of rotation directions, whereas for the case of a finite lattice, the shape of the boundary of the final configuration is indeed a function of the sequence of rotation directions. An analytical model is used to show that the stiffness of the lattice structure can be tuned through folding transition. The authors also investigate the evolution of the structure’s band gaps (i.e., frequency ranges of strong wave attenuation) in folding process using the finite element method.